First published:
Journal of Creation
16(3):11–14
December 2002

Browse this issue

Subscribe to Journal of Creation

MOND over dark matter?

by Bill Worraker

The average orbital velocities of planets in the solar system vary as the inverse square root of distance from the Sun. Thus the Earth orbits at about 30 km/s, Mercury at 48 km/s and Pluto at a leisurely 4.7 km/s. This behaviour, an expression of Kepler’s laws of planetary motion, is termed ‘Keplerian’. It arises from Newtonian dynamics, including the inverse-square law of gravitational attraction, together with the fact that most of the mass in the solar system is concentrated very close to the centre in the Sun.

Figure 1. Rotation curve of the galaxy M31 in Andromeda plotted to scale against an optical photograph of the galaxy. Measurements were based on 21-cm radio emission from neutral hydrogen, which extends beyond the visible edge of the galaxy (this hydrogen is not counted in its presumed ‘dark matter’ inventory) (From Begelman and Rees).⁴¹

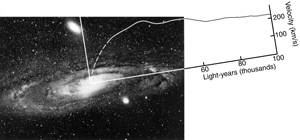
When mid-twentieth century astronomers first investigated galaxy rotations, they expected orbital velocities outside the nuclear bulge to decrease with distance from the centre in Keplerian fashion. Indeed the earliest attempts to obtain rotation curves (plots of orbital velocity against distance from the centre) for spiral galaxies, although subject to large measurement errors, produced results roughly consistent with this expectation. However during the 1970s Vera Rubin and colleagues used the Doppler shifts of spectral lines—mainly optical emission lines from clouds of ionized hydrogen and the 21-cm radio emission line from neutral hydrogen—to establish reliable rotation curves for numerous spiral galaxies.^1–3 The results were both surprising and remarkably consistent: rotation curves in the outer regions of galaxies did not fall with radius in Keplerian fashion. Instead they stayed roughly constant, or even rose slightly towards the outer edges of galaxies. An illustrative rotation curve is shown in Figure 1.

The mass discrepancy problem

This result may be viewed as a discrepancy between galaxy masses inferred dynamically and from their emitted light distributions—the ‘mass discrepancy’ problem. Once generally accepted, it was taken as evidence that galaxies were accompanied by very significant quantities of otherwise undetected or ‘dark’ matter distributed up to and often beyond, their visible boundaries.⁴ This postulated dark matter is important in today’s mainstream cosmology. Modern cosmology proposes a ‘flat’ inflationary big-bang universe in which the effective mass density of the universe consists of dark energy, dark matter and the more familiar visible matter (stars, gas, planets and the like), with the latter only contributing a few percent of the total. Galaxy formation is thought to have begun with density variations in the supposed distribution of dark matter in the early expanding universe. Not only have objects such as white dwarf stars, brown dwarfs, black holes and neutrinos been proposed to account for the dark matter, but also various exotic hypothetical objects including gravitinos, photinos, axions, magnetic monopoles, WIMPs and MACHOs.⁵

But, we ask, is all this really good science? To begin with, the presence of dark matter has recently been called into question in creationist^6,7 and in general astronomical literature.^8–11 The recent detection of faint white dwarf stars allegedly belonging to the Milky Way halo population was hailed as revealing a sample of the elusive dark matter.¹² However, these stars more probably belong to a thick galactic disk population.¹³

Not only this, but have alternative interpretations of the mass discrepancy problem been proposed and only rejected after careful investigation? The answer to this last question proves to be very interesting. As early as 1963 Finzi¹⁴ suggested a distance-based modification of gravity to resolve the mass discrepancy problem for galaxy clusters, but this seems to have received little attention. Then in 1983 the Israeli physicist Moti Milgrom proposed¹⁵ a modification of Newtonian dynamics, known as MOND, designed to reproduce the observed ‘flat’ galaxy rotation curves using only observed distributions of visible matter and reasonable assumptions about mass/light ratios as input data. MOND applies at the very low accelerations which occur in the outer regions of spiral galaxies and in galaxy groups; accelerations are higher in the familiar (inner) region of the solar system. The Newtonian equation for a particle moving under gravity, viz

a = GM / r²

(where a is the radial acceleration, G the universal gravitational constant, M the attracting mass and r the distance from the centre of mass), becomes

a² / a₀ = GM / r²

where a₀ is the critical acceleration level below which MOND applies.¹⁶ This can be viewed as either a modification of the law of inertia or of the law of gravity,¹⁵ the latter being preferred because it involves a less radical modification of recognised physics.¹⁷ Indeed there are still several mysteries surrounding gravity, for example possible shielding of the Sun’s gravity by the Moon during solar eclipses^18,19 and general relativistic ‘frame dragging’, shortly to be investigated by the Gravity Probe B experiment.²⁰ Van Flandern²¹ has reviewed some of the questionable aspects of our understanding of gravity.

Wright, Disney and Thompson²² have suggested a modified (inverse linear) law of gravity beyond a certain distance scale to explain the mass discrepancy problem in galaxies, galaxy clusters and superclusters. Liboff²³ and others have considered similar possibilities too, but McGaugh and de Blok²⁴ insist that acceleration, not distance, is the decisive factor. This is because the mass discrepancy is less severe for high surface brightness (HSB) galaxies than for smaller, low surface brightness (LSB) galaxies where centripetal accelerations fall to extremely low values of order 10^–11 ms^–2.

By using MOND for a single universal value of a₀ , i.e. 1.2 ×10^–10 ms^–2 (about 100 billion times smaller than the acceleration due to gravity at the Earth’s surface), the rotation curves of many galaxies can be reproduced without assuming the presence of appreciable quantities of dark matter!²⁵

Testing MOND predictions

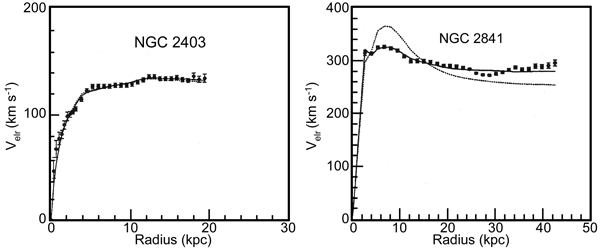
When Milgrom’s ideas were first published, sufficient rotation curves of HSB galaxies were already established that MOND calculations could scarcely be regarded as predictions; they could rather be viewed as parameter-fitting exercises for a₀. Since then, however, rotation curves for LSB galaxies have become available which provide a more stringent test of MOND than the earlier data. The results are remarkably good.^24,26 Furthermore, MOND naturally predicts the Tully-Fisher relation for spiral galaxies,²⁷ the observed correlation between rotation velocities and mass (originally expressed in terms of neutral hydrogen line widths and absolute luminosity) which is often used as a distance indicator. A detailed comparison between MOND and ‘Dark Matter’ predictions for a sample of spiral galaxies with accurately measured rotation curves²⁸ concluded that MOND provided the best available description. This was despite ‘Dark Matter’ being allowed three adjustable model parameters, while in most cases MOND was only allowed one, the mass/light ratio for each galaxy. Figure 2 shows sample MOND fits to measured rotation curves from this paper.

Figure 2. MOND fits to the rotation curves of sample galaxies from Ref. 28 using the value of a₀ given in the text. Dotted curves are one-parameter fits (M/L) while solid curves are two-parameter fits (M/L and distance).

Galaxy rotation is not the only way of testing theories of gravity or inertia. Astronomer Stacy McGaugh (University of Maryland) has set up a MOND web site,²⁹ which includes a page³⁰ listing itemized comparisons of the predictions of MOND and ‘Dark Matter’. These deal with the dynamics of individual elliptical and spiral galaxies of various sizes, motion within galaxy clusters, large scale structure in the universe, gravitational lensing and indeed the spatial structure of the cosmic microwave background radiation. Frequently MOND gives clear predictions where the dark matter hypothesis either has serious fine-tuning problems or gives no prediction. In most cases MOND predictions match the data very well, though sometimes the data is inadequate to provide a clear test. Rubin³¹ briefly alludes to X-ray observations^32,33 which apparently discredit MOND predictions for galaxy clusters. However McGaugh and de Blok²⁴ point out that this data is subject to major uncertainties which preclude a critical test, and that the dark matter interpretation is also problematical, and Sanders³⁴ has shown how MOND neatly predicts the mass-temperature relationship for gas-rich galaxy clusters. Some authors^15,17,35 have noted that the MOND acceleration constant a₀ is of the same order as cH₀ , where c is the speed of light and H₀ the Hubble constant, thus hinting at its possible cosmological significance_.

Gravitational lensing appears to be the least promising area for MOND. However, this is essentially a GR (general relativistic) phenomenon, and MOND has yet to be formulated in GR terms. Thus Binney³⁶ has commented that MOND cannot fairly be tested against such effects. Milgrom³⁵ has recently considered its possible origin in vacuum effects, and Sanders³⁷ has shown how scalar-tensor theories can be constructed which reproduce many MOND predictions. The main objection to MOND is thus summed up by Livio: ‘Milgrom’s conjecture has not gained many supporters, primarily because it has never been developed into a truly complete theory.’³⁸ Future developments could change this.

Why is MOND unpopular?

The answer to our question regarding the interpretation of the mass discrepancy problem is, therefore, that MOND is a very successful, albeit purely phenomenological, alternative to dark matter. It is strongly favoured by Occam’s razor in that it makes clear predictions and is therefore falsifiable, but remains unfalsified. However, rather than being carefully investigated by experts in the field, it has largely been ignored. Those who have commented tend to dismiss it summarily. Thus Rubin says: ‘this possibility must remain as a last resort’³⁹ and ‘Most astronomers prefer to accept a universe filled with dark matter rather than to alter Newtonian gravitational theory.’⁴⁰ Thus it seems that MOND has been ignored not for objective scientific reasons but largely because it implies the existence of much less dark matter than is required by the currently dominant flat, accelerating-universe cosmology which Livio⁵ regards as so beautiful that it ‘has’ to be true.

What interest do creationists have in MOND, a purely mechanistic theory, which was not developed with origins in mind? First, creationist astronomers and cosmologists should be aware of developments which, at least indirectly, impinge on our understanding of the astronomical data relevant to creation. Moreover the remarkably successful predictions of MOND could be pointing to the need for fundamentally new physics at a time when we are hearing that human understanding of the physical universe is nearly complete! MOND exposes deep cracks behind the self-confident façade of modern uniformitarian cosmology and thus reminds us of the fallibility of scientific paradigms, especially when they have been developed in ignorance of the Creator. Recall Isaiah 29:14, which says of those who ignore God: ‘ … the wisdom of the wise will perish, the intelligence of the intelligent will vanish.’

Related articles

• No dark matter found in the Milky Way Galaxy
• Dark matter and a cosmological constant in a creationist cosmology?
• Has ‘dark matter’ really been proven?

Further reading

• Astronomy and Astrophysics Questions and Answers

Related resources

Starlight, Time and the New Physics

by Dr John Hartnett

Written by Dr John Hartnett, this is a bold new answer to the distant starlight issue. Many still doubt the Bible’s clear timescale because, they think, it is impossible for light to have reached the Earth in only a few thousand years from stars that are millions of light-years away. This misconception is often the ultimate stumbling block to a straightforward acceptance of the Bible—even the gospel itself. Understandable by intelligent laypersons, with technical equations and papers put into 111 pages of appendices for the specialist. (High School–Adult) 231 pages.

Hubble, Bubble: Big Bang in Trouble

by Dr John Hartnett

Contrary to the big bang theory’s billions of years, we may actually be seeing a 6,000-years-ago creation right now! Physicist Dr Hartnett argues persuasively that quasars are not massive objects at the universe’s edge, but embryonic galaxies ejected from mature galaxies in our own neighbourhood. (High School–Adult) 50 mins. While the content of this popular, illustrated presentation DVD is the same as one you may have with the same title, it now includes extra features. Not only does it have a new cover design, it also has English sub-titles, has been ‘re-badged’ to feature the CMI logo and contact details and includes a 3-minute promotional segment.

References

1. Rubin, V.C., Bright Galaxies Dark Matters, Springer-Verlag Inc., New York, 1996. Return to text.
2. Rubin, V.C., Ford, W.K., Jr. and Thonnard, N., Rotational properties of 21 Sc galaxies with a large range of luminosities and radii, from NCG 4605 (R = 4 kpc) to UGC 2885 (R = 122 kpc), Ap. J. 238:471–487, 1980. Return to text.
3. Rubin, V.C., Burstein, D., Ford, W.K., Jr. and Thonnard, N., Rotation velocities of 16 Sa galaxies and a comparison of Sa, Sb, and Sc rotation properties, Ap. J. 289:81–104, 1985. Return to text.
4. Dark matter had also been inferred from the large velocity dispersions observed in galaxy clusters by Zwicky and Oort in the 1930s. Return to text.
5. Livio, M., The Accelerating Universe, John Wiley & Sons, Inc., New York, 2000. Return to text.
6. Oard, M. and Sarfati, J., No dark matter found in the Milky Way galaxy, Journal of Creation 13(1):3–4, 1999. Return to text.
7. DeYoung, D.B., Dark matter, CRSQ 36(4):177–182, 2000. Return to text.
8. Lucentini, J.A., Handle on dark matter? Sky and Telescope 103(1):16–17, (News Notes, January 2002), 2002. Return to text.
9. Olling, R.P. and Merrifield, M.R., Luminous and dark matter in the Milky Way, MNRAS 326(1):164–180, 2001. Return to text.
10. Binney, J.J. and Evans, N.W., Cuspy dark matter haloes and the galaxy, MNRAS 327(2), pp. L27–L31, 2001. Return to text.
11. Governato, F., Moore, B., Cen, R., Stadel, J., Lake, G. and Quinn, T., The Local Group as a Test of cosmological models, New Astronomy 2(2):91–106, 1997. Return to text.
12. Oppenheimer, B.R., Hambly, N.C., Digby, A.P., Hodgkin, S.T. and Saumon, D., Direct detection of galactic halo dark matter, Science 292(5517):698–702, 2001. Return to text.
13. Reid, I.N., Sahu, K.C. and Hawley, S.L., High-velocity white dwarfs: thick disk, not dark matter, Ap. J. 559(2):942–947, 2001. Return to text.
14. Finzi, A., On the validity of Newton’s Law at a long distance, MNRAS 127:21–30, 1963. Return to text.
15. Milgrom, M., A modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis, Ap. J. 270:365–370, A modification of the Newtonian dynamics: implications for galaxies, 371–383 and A modification of the Newtonian dynamics: implications for galaxy systems, 384–389 (referred to as papers I, II and III where necessary), 1983. Return to text.
16. There is a ‘transition range’ of accelerations characterized by a~a₀ where an interpolation function, not prescribed a priori, is required to match the conventional and MOND regimes, but MOND predictions do not seem to depend critically on the form chosen for this function. Return to text.
17. Bertin, G., Dynamics of Galaxies, Cambridge University Press, 2000. Return to text.
18. Wang, Q.S., Yang, X.S., Wu, C.Z., Guo, H.G., Liu, H.C. and Hua, C.C., Precise measurements of gravity variations during a total solar eclipse, Phys. Rev. D 62:041101, (pp. 1–3), 2000. Return to text.
19. Unnikrishnan, C.S., Mohapatra, A.K. and Gillies, G.T., Anomalous gravity data during the 1997 total solar eclipse do not support the hypothesis of gravitational shielding, Phys. Rev. D 63:062002, (pp. 1–4), 2001. Return to text.
20. See <einstein.stanford.edu/>, 16 July 2002. Return to text.
21. <www.metaresearch.org/cosmology/gravity/possiblenewpropertiesofgravity.asp>, 16 July 2002; see also Van Flandern, T., Possible new properties of gravity Ap&SS 244:249–262, 1996. Return to text.
22. Wright, A.E., Disney, M.J. and Thompson, R.C., Universal gravity: was Newton right? Proc. ASA 8(4):334–338, 1990. Return to text.
23. Liboff, R.L., Generalized force law and hidden mass, Ap. J. 397:L71–L73, 1992. Return to text.
24. McGaugh, S.S. and de Blok, W.J.G., Testing the hypothesis of modified dynamics with low surface brightness galaxies and other evidence, Ap. J. 499:66–81, 1998. Return to text.
25. Sanders, R.H. and Verheijen, M.A.W., Rotation curves of Ursa Major galaxies in the context of modified Newtonian dynamics, Ap. J. 503:97, 1998. Return to text.
26. de Blok, W.J.G. and McGaugh, S.S., Testing modified Newtonian dynamics with low surface brightness galaxies: rotation curve fits, Ap. J. 508:132–140, 1998. Return to text.
27. Tully, R.B. and Fisher, J.R., A New method of determining distances to galaxies, Astr. Ap. 54:661–673, 1977. Return to text.
28. Begeman, K.G., Broeils, A.H. and Sanders, R.H., Extended rotation curves of spiral galaxies: dark haloes and modified dynamics, MNRAS 249:523–537, 1991. Return to text.
29. <www.astro.umd.edu/~ssm/mond/>, 7 June 2002. Return to text.
30. <www.astro.umd.edu/~ssm/mond/mondvsDM.html>, 7 June 2002. Return to text.
31. Rubin, Ref. 1, pp. 137, 138. Return to text.
32. Buote, D.A. and Canizares, C.R., X-ray constraints on the shape of the dark matter in five Abell clusters, Ap. J. 400(2):385–397, 1992. Return to text.
33. Buote, D.A. and Canizares, C.R., Geometrical evidence for dark matter: X-ray constraints on the mass of the elliptical galaxy NGC 720, Ap. J. 427(1):86–111, 1994. Return to text.
34. Sanders, R.H., A Faber-Jackson relation for clusters of galaxies: implications for modified dynamics, Astr. Ap. 284,L31–L34, 1994. Return to text.
35. Milgrom, M., The modified dynamics as a vacuum effect, <arxiv.org/astro-ph/pdf/9805346>, 1999 (or Phys. Lett. A 253:273–, 1999). Return to text.
36. Binney, J.J., private communication, 2002. Return to text.
37. Sanders, R.H., A Stratified Framework for Scalar-Tensor Theories of Modified Dynamics, Ap. J. 480:492–, 1997. Return to text.
38. Livio, Ref. 5, p. 90. Return to text.
39. Rubin, Ref. 1, p. 115. Return to text.
40. Rubin, Ref. 1, p. 125. Return to text.
41. Begelman, M.C. and Rees, M.J., Gravity’s Fatal Attraction: Black Holes in the Universe, Scientific American Library, W.H. Freeman and Co., ISSN 1040–3213: no. 58, 1996. Return to text.